PLANE GEOMETRY VS SOLID GEOMETRY: UNDERSTANDING THEIR DIFFERENCES AND INTERPLAY
Discover the fascinating world of plane geometry vs solid geometry: understanding their differences and interplay. This insightful book will help you to better understand geometric concepts.
Take a trip through the fascinating world of geometry. This is a beautiful and logical realm with roots that go back to the Greeks. This mathematical domain contains two branches that are intertwined: solid geometry and plane geometry.
The plane geometry course, which focuses on 2D shapes, and the solid geometry course, which focuses on 3D shapes, explores the properties, measurements, and relationships between shapes. This opens up a world of patterns and forms.
DISCOVERING THE WORLD OF PLANE SHAPES
Plane geometry is aptly called for the fact that it only has two dimensions: length and width. It contains a variety of flat figures such as lines, circles, and polygons. Each figure is defined by its edges and angles.
The polygon is a 2D figure that’s made up of converging straight-line segments (sides). The number of sides determines the sum of its interior angle.

Take into consideration a triangle, a polygon having three sides and a total sum of 180 degrees for its internal angles. A square with four sides has a total sum of 360 degrees. Polygons also have symmetry, regularity, and convexity.
Plane geometry goes beyond simple shape identification by introducing concepts such as perimeter (the total length of the edges on a form) and area (the space enclosed within a particular shape). These measurements have many practical applications. They can be used to estimate paint quantities on a wall or calculate fence lengths in a yard.
EXPLORING THE UNIVERSE OF SOLID OBJECTS
Solid geometry is the upper floors in a geometric building that expands into the third dimension, depth. The spheres and cubes are defined by their surface area, volume, and spatial relationship. The study of 3D transformations is fascinating. It’s like watching animations. Objects can move, rotate, and reflect. They can also scale up or down. These transformations reveal the spatial and symmetries of 3D objects.
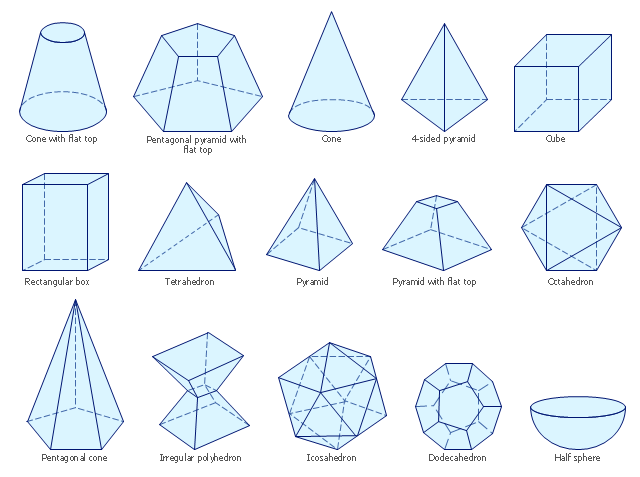
Solid geometry also includes volumetric calculations to determine the area occupied by 3D shapes. By multiplying the length, width, and height of a cube, one may determine its volume. This knowledge is useful in engineering. It can be used to assess storage capacity or analyze structural efficiency.
THE DANCE BETWEEN PLANE AND SOLID GEOMETRY
The interplay between solid and plane geometry shapes our understanding of 3D and 2D space. Their dynamic relationship provides insights into geometric transformations, intersections, and mathematical properties.
Slice a cone using a plane to create different conic sections, such as circles, ellipses, or parabolas. Similar to 3D shape slicing, 2D cross sections are created by 3D shape slicing. These 2D cross-sections are vital for engineering and architecture.

The connection between solid and plane geometry is crucial for computer graphics and animation. This connection enhances mathematic understanding and problem solving skills across disciplines.
CONCLUSION
A flat map may look different from a globe at first. Just as a flat map can be compared to a globe, 2D shapes provide insight into 3D forms. Understanding the relationship between these two forms gives us valuable tools for understanding, measuring, and navigating our complex world.
Recognizing the interplay of plane and solid geometries enriches our mathematics understanding. The beauty of geometry is not just in its depth, but also in the intricate connections between disciplines.